Introduction Section
This test is the multivariate analog of the two-sample t-test in univariate statistics. These two sample tests, in both cases, are used to compare two populations. Two populations may correspond to two different treatments within an experiment.
For example, in a completely randomized design, the two treatments are randomly assigned to the experimental units. Here, we would like to distinguish between the two treatments. Another situation occurs where the observations are taken from two distinct populations. In either case, there is no pairing of the observations.
Example 7-12: Swiss Bank Notes Section
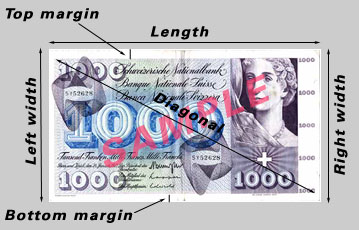
An example where we are sampling from two distinct populations occurs with 1000 franc Swiss Bank Notes.
- The first population is the population of Genuine Bank Notes
- The second population is the population of Counterfeit Bank Notes
While the diagram shows a more recent version issue of the 1000 franc note, it depicts the different measurement locations taken in this study. For both populations of banknotes the following measurements were taken:
- Length of the note
- Width of the Left-Hand side of the note
- Width of the Right-Hand side of the note
- Width of the Bottom Margin
- Width of the Top Margin
- Diagonal Length of Printed Area
Objective: To determine if counterfeit notes can be distinguished from genuine Swiss banknotes.
This is essential for the police if they wish to use these kinds of measurements to determine if a banknote is genuine or not and help solve counterfeiting crimes.
Before considering the multivariate case, we will first consider the univariate case...