10.2.1 - ANOVA Assumptions

Assumptions for One-Way ANOVA Test Section

There are three primary assumptions in ANOVA:

  1. The responses for each factor level have a normal population distribution.
  2. These distributions have the same variance.
  3. The data are independent.
Note! Violations to the first two that are not extreme can be considered not serious. The sampling distribution of the test statistic is fairly robust, especially as sample size increases and more so if the sample sizes for all factor levels are equal. If you conduct an ANOVA test, you should always try to keep the same sample sizes for each factor level.

A general rule of thumb for equal variances is to compare the smallest and largest sample standard deviations. This is much like the rule of thumb for equal variances for the test for independent means. If the ratio of these two sample standard deviations falls within 0.5 to 2, then it may be that the assumption is not violated.

Example 10-1: Tar Content Comparisons Section

Recall the application from the beginning of the lesson. We wanted to see whether the tar contents (in milligrams) for three different brands of cigarettes were different. Lab Precise and Lab Sloppy each took six samples from each of the three brands (A, B and C). Check the assumptions for this example.

Answer

Lab Precise

  1. The sample size is small. We should check for obvious violations using the Normal Probability Plot.

    The graph shows no obvious violations from Normal, but we should proceed with caution.

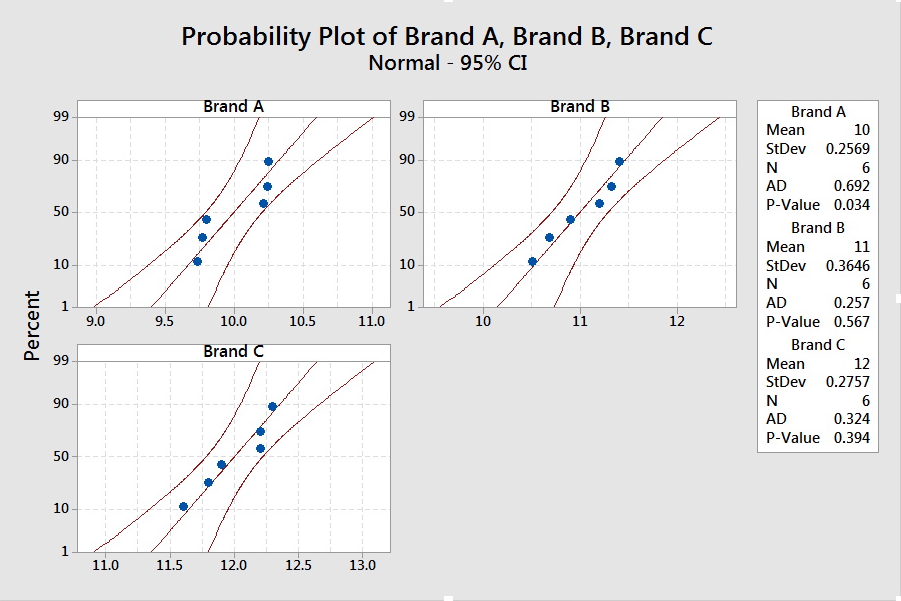
    Normal probability plot of Brand A, B, and C
  2. The summary statistics for the three brands are presented.

    Descriptive Statistics: Precise Brand A, Precise Brand B, Precise Brand C

    Variable

    Mean

    StDev

    Precise Brand A

    10.000

    0.257

    Precise Brand B

    11.000

    0.365

    Precise Brand C

    12.000

    0.276

    The smallest standard deviation is 0.257, and twice the value is 0.514. The largest standard deviation is less than this value. Since the sample sizes are the same, it is safe to assume the standard deviations (and thus the variances) are equal.

  3. The samples were taken independently, so there is no indication that this assumption is violated.

Lab Sloppy

  1. The sample size is small. We should check for obvious violations using the Normal Probability Plot.

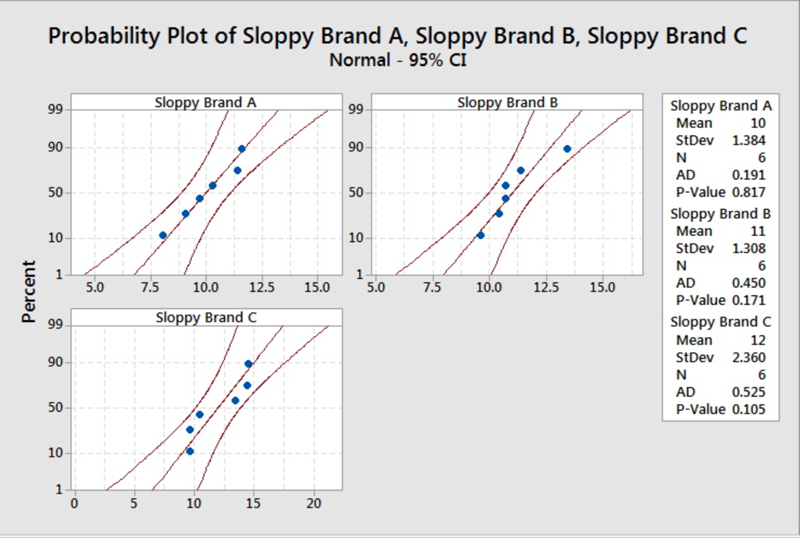
    Normal probability plots for Sloppy Brands A, B and C.
  2. The summary statistics for the three brands are presented.

    Descriptive Statistics: Sloppy Brand A, Sloppy Brand B, Sloppy Brand C

    Variable

    Mean

    StDev

    Sloppy Brand A

    10.000

    1.384

    Sloppy Brand B

    11.000

    1.308

    Sloppy Brand C

    12.000

    2.360

    The smallest standard deviation is 1.308, and twice the value is 2.616. The largest standard deviation is less than this value. Since the sample sizes are the same, it is safe to assume the standard deviations (and thus the variances) are equal.

  3. The samples were taken independently, so there is no indication that this assumption is violated.