3.2.3 - Minitab: Binomial Distributions

Minitab 18

Minitab®  – Finding Binomial Probabilities using Minitab

Let’s walk through how to calculate the probability of 1 out of 3 crimes being solved in the FBI Crime Survey example.

Recall in that example, \(n=3\), \(p=0.2\).

Using Minitab, calculate \(P(X=1)\):

  1. From the Minitab menu select Calc > Probability Distributions > Binomial
  2. A dialog box (below) will appear. Enter 3 into the Number of Trials box and 0.2 into the Event Probability box.
  3. Choose Probability .
  4. Choose the Input Constant Box and enter 1.
  5. Choose OK .

Minitab binomial window

The result should be the same probability of 0.384 we found by hand.

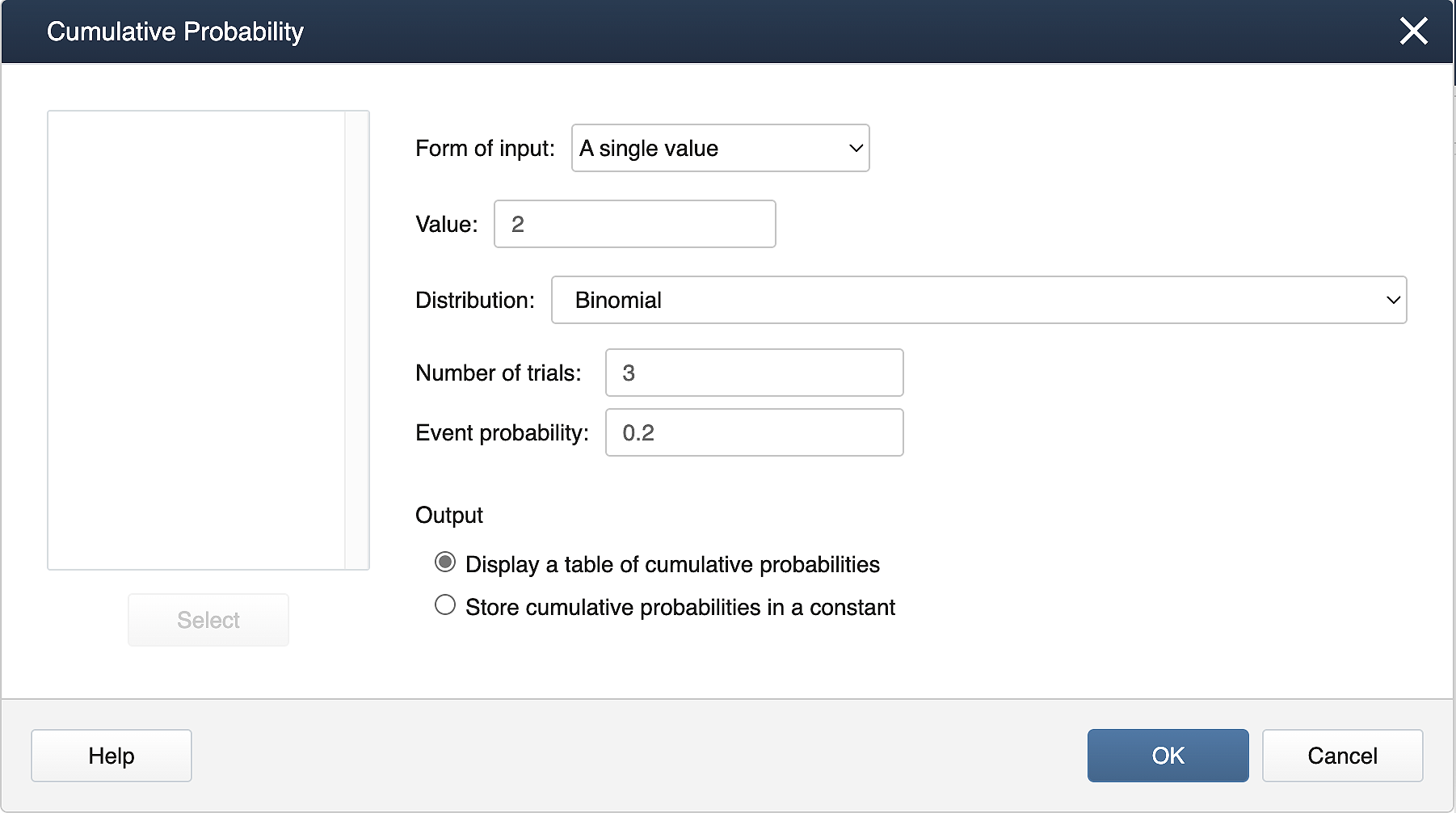
Suppose we want to find \(P(X\le 2)\). We can use Minitab to find this cumulative probability.

  1. From the Minitab menu select Calc > Probability Distributions > Binomial
  2. Enter in 3 and 0.2 as above.
  3. Choose Cumulative Probability .
  4. Choose Input Constant and enter 2.
  5. Choose OK .

The result should be \(P(X\le 2)=0.992\).

Note! While using Minitab is quicker, you may be expected to compute these probabilities by hand on assignments.

Note! Section

Depending on if you have the desktop version or cloud version of MINITAB, you will find minor differences in the order of the steps.

Binomial with Minitab

  1. Suppose X follows a binomial distribution with 𝑛=3 and 𝑝=0.2. Calculate 𝑃(𝑋=1).  

    Calc > Probability Distributions > Probability Density. Fill the dialog box as shown below.

    Minitab Probability Density window

     

    Binomial with n= 3 and p = 0.2

    x

    P(X=x)

    1

    0.383

  2. Suppose X follows a binomial distribution with 𝑛=3 and 𝑝=0.2. Calculate P(𝑋≤2).

    Calc > Probability Distributions > Cumulative Distribution Function. Fill the dialog box as shown below.   
     

     

    Binomial with n= 3 and p = 0.2

    x

    P(X≤x)

    2

    0.992