Minitab® – Procedure
You may need to find a P-value if you are using the P-value approach to conduct a hypothesis test that uses a t-statistic.
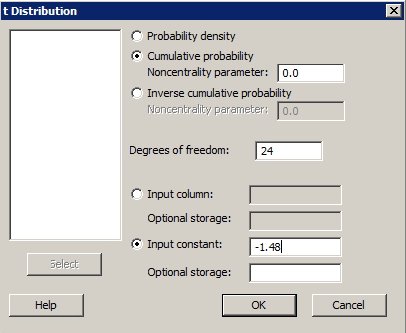
- Select Calc >> Probability Distributions >> t ...
- Click the button labeled 'Cumulative probability'.
- Type the number of degrees of freedom in the box labeled 'Degrees of freedom'.
- Click the button labeled 'Input constant'. In the box, type the test statistic for which you want to find the associated cumulative probability.
- Select OK. The probability that a t-distributed random variable with this number of degrees of freedom is less than or equal to the test statistic will appear in the session window.
- The P-value is this probability for a lower-tail test or one minus this probability for an upper-tail test. For a two-tail test multiply the one-tail probability by two.
Example
The US National Research Council currently recommends that females between the ages of 11 and 50 intake 15 milligrams of iron daily.
Is there evidence that the population of American females is, on average, getting less than the recommended 15 mg of iron? That is, should we reject the null hypothesis H0: μ = 15 against the alternative HA: μ < 15?
The iron intakes (irondef.txt) of a random sample of 25 such American females yielded a t-statistic of -1.48.
If we were interested in calculating the test at the α = 0.05 level, what is the appropriate P-value to which we should compare the t-statistic?
Minitab Dialog Box
Sample Minitab Output
Cumulative Distribution Function
Student's t distribution with 24 DF
| x | P ( X ≤ x) |
|---|---|
| -1.48 | 0.0759408 |